Chapter 5 Continuity and Differentiability Miscellaneous Exercise

Chapter 5 Continuity and Differentiability Miscellaneous Exercise

Question 1.
(3x2 - 9x + 5)9
Answer:
Let y = (3x2 - 9 + 5)2
Differentiating both sides wr.t. x
$\frac{dy}{dx}$ = 9(3x2 - 9x + 5)8 . $\frac{d}{dx}$ (3x2 - 9x + 5)
= 9(3x2 - 9x + 5)8.(6x - 9)
= 9(6x - 9) (3x2 - 9x + 5)8
= 9 × 3(2x - 3) (3x2 - 9x + 5)8
= 27(2x - 3) (3x2 - 9x + 5)8

Question 2.
sin3 x + cos6 x
Answer:
Let y = sin3 x + cos6 x
Differentiating both sides w.r.t. x
$\frac{dy}{dx}$ = 3 sin2 x . $\frac{d}{dx}$ (sin x) + 6 cos5 x . $\frac{d}{dx}$ (cos x)
= 3 sin2 x cos x + 6 cos5 x (- sin x)
= 3 sin2 x cos x - 6 cos5 x sin x
= 3 sin x cos x (sin x - 2 cos4 x)

Question 3.
(5x)3 cos 2x
Answer:
Let y = (5x)3 cos 2x
Taking logarithm on both sides, we get
log y = 3 cos 2x log (5x)
Now, differentiating both sides w.r.t x

Question 4.
sin-1 (x√x), 0 ≤ x < 1
Answer:
Let y = sin-1 (x√x) ⇒ y = sin-1 (x)3/2
Differentiating both sides w.r.t. x

Question 5.
$\frac{{\cos }^{-1}\frac{x}{2}}{\sqrt{2x+7}}$, - 2 < x < 2
Answer:

Question 6.
cot-1$\left[\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right]$, 0 < x < $\frac{\pi }{2}$
Answer:

Question 7.
(log x)log x, x > 1
Answer:
Let y = (log x)log x
log y = log x.{log (log x)}
Now, differentiating both sides w.r.t. x

Question 8.
cos (a cos x + b sin x), for some constants a and b.
Answer:
Let y = cos (a cos x + b sin x)
Differentiating both sides w.r.t. x
$\frac{dy}{dx}$ = - sin(a cos x + b sin x) × $\frac{d}{dx}$(a cos x + b sin x)
⇒ $\frac{dy}{dx}$ = - sin(a cos x + b sin x) × {- a sin x + b cos x}
Thus, $\frac{dy}{dx}$ = (a sin x - b cos x) × (a cos x + b sin x)

Question 9.
(sin x - cos x)(sin x - cos x), $(\frac{\pi }{4}<x<\frac{3\pi }{4})$
Answer:
Let y = (sin x - cos x)(sin x - cos x)
Taking logarithm on both sides
log y = (sin x - cos x) log (sin x - cos x)
Now, differentiating both sides w.r.t. ‘x’

Question 10.
xx + xa + ax + aa, for same fixed a > 0 and x > 0
Answer:
Let y = xx + xa + ax + aa
Differentiating both sides w.r.t. x

Question 11.
xx2 - 3 + (x - 3)x2 for x > 3
Answer:
Let y = xx2 - 3 + (x - 3)x2
and u = xx2 - 3, y = (x - 3)x2
∴ y = u + v
Differentiating both sides w.r.t. x
$\frac{dy}{dx}=\frac{du}{dx}-\frac{dv}{dx}$ ........ (1)
Now, taking logarithm on both sides of u = xx2 - 3
log u = (x2 - 3)log x
Differentiating both sides of w.r.t. x
Now, taking logarithm on both sides of u = xx2 - 3
log u = (x2 - 3)log x
Differentiating both sides of w.r.t. x

Again, taking logarithm on both sides of v = (x - 3)x2
log v = x2log(x - 3)
Differentiating both sides w.r.t. z

Question 12.
If y = 12(1 - cos t), x = 10(t - sin t), - $\frac{\pi }{2}$ < t < $\frac{\pi }{2}$, then find $\frac{dy}{dx}$.
Answer:
We have,
y = 12(1 - cos t) and x = 10(t - sin t)
Differentiating x and y wr.t. t
$\frac{dy}{dt}$ = 12{0 - (- sin t)} = 12 sin t

Question 13.
If y = sin-1x + sin-1 $\sqrt{1-x^2}$, 0 < x < 1 then find $\frac{dy}{dx}$.
Answer:
Given, y = sin-1 x + sin-1 $\sqrt{1-x^2}$
Putting sin-1 x = θ ⇒ x = sin θ
∴ y = θ + sin-1 ($\sqrt{1-{\sin }^2\theta }$)
⇒ y = θ + sin-1 (cos θ)
⇒ y = θ + sin-1 $\left[\sin (\frac{\pi }{2}-\theta )\right]$
⇒ y = θ + $\frac{\pi }{2}$ - θ ⇒ y = $\frac{\ne }{2}$
Differentiating both sides w.r.t. x
$\frac{dy}{dx}$ = 0

Question 14.
If x$\sqrt{1+y}$ + y$\sqrt{1+x}$ = 0 for - 1 < x < 1, then prove that $\frac{dy}{dx}=-\frac{1}{(1+x{)}^2}$.
Answer:
We have x$\sqrt{1+y}$ + y$\sqrt{1+x}$ = 0
⇒ x$\sqrt{1+y}$ = - y$\sqrt{1+x}$
Squaring both sides
(x$\sqrt{1+y}$)2 = (- y$\sqrt{1+x}$)2
⇒ x2(1 + y) = y2(1+ x)
⇒ x2 + x2y = y2 + xy2
⇒ x2 + x2y - y2 - xy2 = 0
⇒ x2 - y2 + x2y - xy2 = 0
⇒ (x - y) (x + y) + xy(x - y) = 0
⇒ (x - y) {(x + y) + xy} = 0
⇒ x + y + xy = 0 [∵ (x - y) ≠ 0 or x ≠ y]
⇒ y(1 + x) - x ⇒ y = -$\frac{x}{1+x}$
Differentiating both sides w.r.t. x

Question 15.
If (x - a)2 + (y - b)2 = c2 for some c > 0, prove that $\frac{{\{1+{\left(\frac{dy}{dx}\right)}^2\}}^{3/2}}{\frac{d^{2}y}{d{x}^2}}$, is a constant independent of a and b.
Answer:
We have, (x - a)2 + (y - b)2 = c2 ....... (1)
Differentiating both sides w.r.t. x
2(x - a) + 2(y - b).$\frac{dy}{dx}$ = 0
⇒ (x - a) + (y - b) $\frac{dy}{dx}$ = 0 .....(2)
Again, differentiating both sides w.r.t. x

For equation (3) and (4) putting values of (y - b) and (x - a) in equation (1)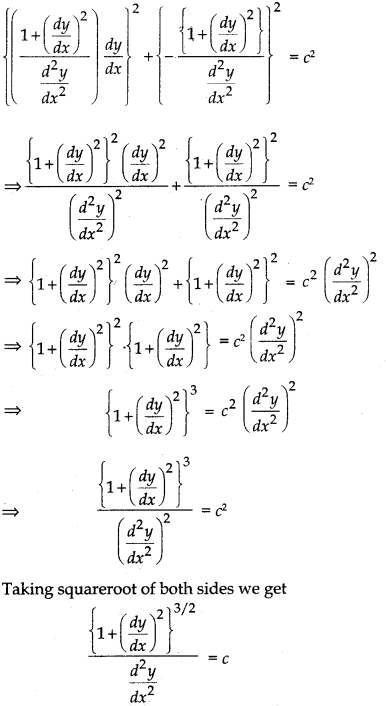

which is independent of a and b.
Hence Proved.

Question 16.
If cos y = x cos (a + y), with cos a ≠ ±1, prove that $\frac{dy}{dx}$ = $\frac{{\cos }^2(a+y)}{\sin a}$.
Answer:
We have, cos y = x cos (a + y)
⇒ $\frac{\cos y}{\cos (a+y)}$ = x
Differentiating both sides w.r.t. x

Question 17.
If x = a(cos t + t sin t) and y = a(sin t - t cos t) then find $\frac{d^{2}y}{d{x}^2}$.
Answer:
We have, x = a(cos t + t sin t)
and y = a(sin t - t cos t)
Differentiating both sides w.r.t t

Question 18.
If f(x) = |x|3, show that f”(x) exists for all real x and find it.
Answer:
Let f(x) = x3
If x > 0, |x| = x then f(x) = x3
Differentiating w.r.t. x
$\frac{d}{dx}$ [f(x)] = $\frac{d}{dx}$ (x3)
⇒ f'(x) = 3x2
Again, differentiating w.r.t. x
f’(x) = 6x ... (1)
Thus f”(x) exists.
When x < 0, |x| = - x
∴ f(x) = |x|3 = (- x3) = - x3
∴ f(x) = - x3 differentiating w.r.t. x
f'(x) = - 3x2
Again, differentiating w.r.t. x
f''(x) = - 6x ..... (2)
Thus, f”(x) exists
From (1) and (2), we have
f'(x) = 6|x|
Hence, it is proved that f''(x) exists.
Hence proved

Question 19.

Using mathematical induction prove that $\frac{d}{dx}$(xn) = nxn - 1 for all positive integers n.
Answer:
We have, $\frac{d}{dx}$(xn) = nxn - 1
Let P(n): $\frac{d}{dx}$(xn) = nxn - 1
Putting n = 1
P(1): $\frac{d}{dx}$(x1) = 1.x1 - 1 = 1.x0 = 1.1 = 1
Thus, given function is true for n = 1 i.e. true for P(1)
Let statement is true for n = k, then
P(k): $\frac{d}{dx}$(xk) = kxk - 1
Now, we will prove that statement is true for n = k + 1
P(k + 1): $\frac{d}{dx}$(xk + 1) = (k + 1)xk
L.H.S. = $\frac{d}{dx}$ (xk + 1) = $\frac{d}{dx}$ (x.xk)
= 1.xk + x.(kxk - 1)
(∵ $\frac{d}{dx}$(xk+1) = (k + 1)xk
= xk + k.xk
= xk (k + 1) = (k + 1)xk = R.H.S.
∴ Statement is true for n = k + 1
Thus, by mathematical induction principle, statement is true for positive integer numbers.

Question 20.
Using the fact that sin (A + B) = sin A cos B + cos A sin B and the differentiation obtain the sum formula for cosines.
Answer:
We have
sin (A + B) = sin A cos B + cos A sin B ..... (1)
Let A and B are function of t then differentiating both sides w.r.t. t
$\frac{d}{dt}$[sin (A + B)] = $\frac{d}{dt}$[sin A cos B + cos A sin Bl
L.H.S. = $\frac{d}{dt}$[sin (A + B)]

⇒ cos (A + B) = cosA cos B - sin A sin B
Thus, cos (A + B) cos A cos B - sin A sin B

Question 21.
Does there exist a function which is continuous everywhere but not differentiable at exactly two points? Justify your answer.
Answer:
Yes, such function exists which is continuous at each point but not differentiable only at two parts. e.g. function f(x) = |x| + |x - 1|, x ∈ R is continuous at all points but not differentiable at x = 0 and x = 1. Since |x|,is not differentiable at x = 0 similarly |x - 1| is not differentiable at x = 1. Thus, function is not differentiable at two points x = 0, x = 1 whereas it is continuous at all points (where x ∈ R).
Similarly, function f(x) = |x - 2| + |x - 3| x ∈ R, is continuous at all points but not differentiable at x = 2, x = 3.

Question 22.
If y = $\left|\begin{array}{ccc}f\left(x\right)& g\left(x\right)& h\left(x\right)\\ l& m& n\\ a& b& c\end{array}\right|$, prove that $\frac{dy}{dx}=\left|\begin{array}{ccc}{f}^{\prime }\left(x\right)& g^{\prime }\left(x\right)& h^{\prime }\left(x\right)\\ l& m& n\\ a& b& c\end{array}\right|$
Answer:
We have,

= f(x) (mc - bn) - g(x) (lc - an) + h(x) (lb - am)
⇒ y = (mc - nb) f(x) + g(x) (na - lc) + hx(lb - ma)
Differentiating w.r.t. x

Question 23.

If y = ea cos-1 x - 1 ≤ x ≤ 1, show that
(1 - x2)$\frac{d^{2}y}{d{x}^2}$ - x$\frac{dy}{dx}$ - a2y = 0
Answer:
We have
y = ea cos-1 x
Differentiating w.r.t. x

सभी प्रकार के नोट्स TOPPRS.IN पर FREE उपलब्ध है !